The Best-Kept Secret in Gifted Education: Above-Level Testing

The secret of above-level testing is really not much of a secret. It’s used extensively at universities that have centers for gifted education. Unfortunately, it’s not used much by schools. This secret is hiding in plain sight!

What is above-level testing and how can it be used?

Let’s answer the second question first. Above-level testing is useful for decisions about:

1. Identifying a student for a gifted program
2. Determining what a student is ready to learn next
3. Deciding whether or not a student is ready for subject-matter acceleration
4. Deciding whether or not a student is ready to skip a grade

“Above-level testing” is exactly what it sounds like: Give a younger student a test that was developed for older students. This idea was pioneered exactly one hundred years ago by Dr. Leta Hollingworth, sometimes called the “mother” of gifted education. This concept was fully developed by Dr. Julian Stanley in the 1970s when he devised the “Talent Search” in which 7th and 8th graders took the college admissions exam, the SAT. Fast forward to 2016, and above-level testing is used extensively in outside-of-school programs for gifted students. In fact, hundreds of thousands of students around the world take above-level tests each year as part of university-based talent searches, such as the one offered by the Belin-Blank Center. Some of these tests used are the SAT, ACT, Explore (recently discontinued), and I-Excel. Unfortunately, above-level tests are not used extensively in typical school gifted programs; we would like to change that!

Academically talented students tend to perform extremely well on tests developed for their own age group. They do so well that they get everything (or almost everything) right, and we don’t really know what the extent of their talents might be. Psychologists call this “hitting the ceiling” of the test. Think of it like a yardstick: The grade-level “yardstick” measures only 36 inches. If the student is 40 inches tall, we can’t measure that accurately using only the grade-level yardstick. What we need is a longer yardstick, and a harder test. An above-level test, one that is developed for older students, provides that longer yardstick and successfully raises the ceiling for that talented student.

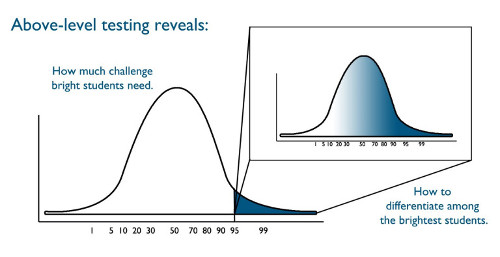
The advantages of above-level testing include differentiating between “talented” and “exceptionally talented” students. In the figure above, the bell curve on the left shows a typical group of students. A few students earn very high scores (at the 95th percentile or above when compared to their age-mates). These are the students who “hit the ceiling” of the grade-level test. If we give that group of students a harder test, an above-level test that was developed for older students, voila! we see a new bell curve (the one on the right). The harder test spreads out the scores of the talented students and helps us to differentiate the talented from the exceptionally talented students.

What does this matter? Knowing how students performed on an above-level test helps us to give the students, their families and their educators better advice about the kinds of educational options the students might need. For example, does this student need educational enrichment? Would that student benefit from moving up a grade level or two in math? Would another student benefit from grade-skipping? Organizations such as the Belin-Blank Center who have used above-level testing for years have developed rubrics to help educators and parents understand the student’s above-level test scores and relate them to appropriately challenging educational options. In just one or two hours of testing, we are able to get important information about the student’s aptitudes, which allows us to make good recommendations about the types of educational challenges the student needs.

We at the Belin-Blank Center are thrilled to be able to provide educators with specific information about your students via the in-school testing option for I-Excel, an above-level test for talented 4th – 6th graders. For more information about how this could work in your school, visit I-Excel or Belin-Blank Center, or contact ann-shoplik@uiowa.edu.

Permission Statement

This article is reprinted with permission from the Belin-Blank Center and is used here with permission.

This article is provided as a service of the Davidson Institute for Talent Development, a 501(c)3 nonprofit dedicated to supporting profoundly gifted young people 18 and under. To learn more about the Davidson Institute’s programs, please visit www.DavidsonGifted.org.